More Probability Distributions of Tides

David Pugh, in Sea Level Science introduces probability distribution diagrams as an analytical tool.  The beeswarm plots from a couple of posts ago are another way of representing distributions along one axis.  The idea is to plot a span of data (or in my case, usually predictions) along a single axis.

What does a probability distribution tell us?  It can give us an idea how many hours per day an organism in a particular zone will be exposed to the air.  It would be more interesting to know the tides of exposure to sunlight.  For corals this is essential.  David Pugh says that the S2 harmonic constituent can give us an idea without graphs.  This is a subject for a future project.

For how, the following is an effort from several months back, breaking down the probability distributions of heights month by month, for San Francisco's venerable tide gauge (thought these may be predictions).  I wonder what this has to say in comparison with St. Petersburg, Florida's beeswarm plot.  Obviously, it's impossible to compare them well using these two distinctly different methods.  The top row shows frequency distributions for January, February, and March, and so on in the other rows; all graphs are from predictions. 

One thing I noticed was a similarity of the distributions for June and December, around times of Solstices.  The same may be said for the months of the Equinoxes, March and September.  I now want to see another graph, the specific one-day distributions for the exact days of Solstices and Equinoxes.  Other parameters, like apogee and perigee, perihelion and aphelion, lunar declination have important effects.

Comparing Two Sites (Full year of predictions for each)

San Simeon is on the exposed California Coast. 
Port Chicago some 30 miles up the Northern Reach of San Francisco Bay, in Suisun Bay.

Months of Solstices and Equinoxes, 

Chuuk Lagoon

 

December

March

June

 

September

 

Equinoxes are in March and September.  Solstices are in December and September.  Why do the Equinoxes resember one another?  Why do the Solstices resemble one another?  ???  

 

Elkhorn Slough December, March, June, and September

The differences between Elkhorn Slough and Chuuk Lagoon are notable.

March

December

June

September

 

 

 

 

 

 

 

 

 

 

 

Another distribution diagram: a continuous distribution curve.

 This time, each month is traced in a different color.  This is experimental; I did not keep a record of the colors for each month!

 And another way to represent similar data, with transparent fill:

Redwood City Tide Station

This morning I visited the Redwood City Tide Station.  Awesome.

The business end.

A Beeswarm plot, for St. Petersburg, Florida

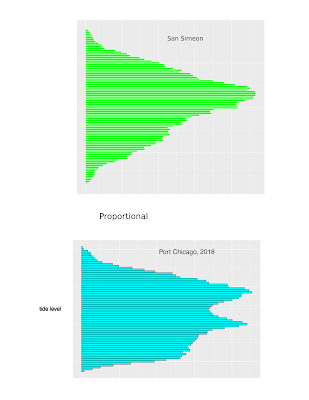
I'll try any kind of graph.  Distribution frequency plots are described in Pugh's 1984 Sea Level Science.  I saw a beeswarm plot, don't remember where; it seemed an interesting way to do a distribution frequency plot.  

In a beeswarm plot, data is plotted along a single axis, in this case, tide water levels.  No overlapping points are allowed; each overlapping point is "jittered" off to one side of the other point(s) at the  

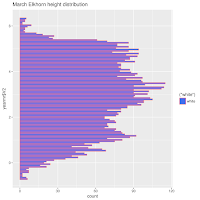
Are beeswarm plots informative?  i tried.  As usual I had to fiddle (speaking of which, a "violin plot" does something similar.  Perhaps I'll try one).  The "beeswarm" package for R makes production of these plots pretty easy.  My first attempt suggested to me that a smaller amount of data might be more manageable. Eventually, something like this appeared, for St Petersburg, FL:

A first approximation: Predictions for June 17--28 

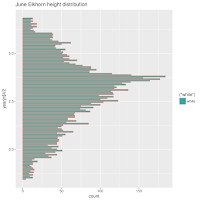
Does the Moon figure into the distribution of water levels.  I think this information is useful when considering intertidal zonation.  The following is a beeswarm plot for  hourly predictions for June, from New Moon to First Quarter June 17--26).  What, then, is this prominaent horizontal feature

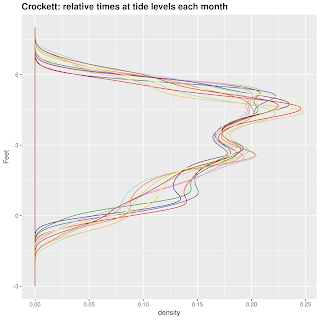
 Comparing with a plot for a couple of these days

Below, the same plot has been placed together with a plot of the tide at St Petersburg for two days in this period of time.  The hold ups seem to be encoded as the horizontal line

 

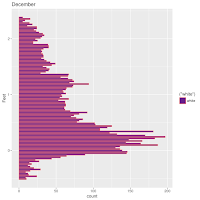
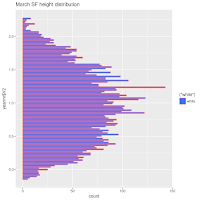
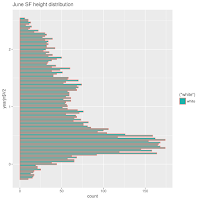
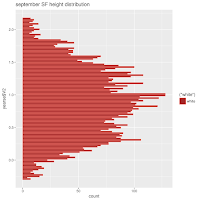
A Month at a Time

As a first approximation, I wondered whether it would be useful to divide time into quarter moons.  The first reason: a useful number of points plotted on a single vertical beeswarm axis looks stupidly cluttered.  Maybe it would be easier to plot short periods of time, like a month?  No, a month was waaay to cluttered.  A week looked alright.

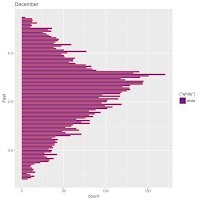
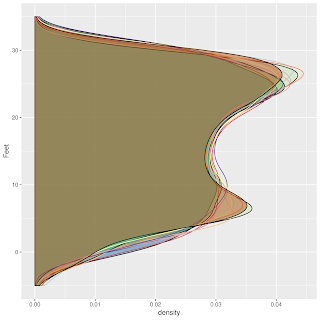
 A month at a time 

So I used quarter moons.  I have some misgivings about this choice.  Next time I may CENTER the time division on a full moon, quarter moon, or new moon.  Here, while it looks interesting, I'm pretty sure that much information about the actual full or new moon's influence.

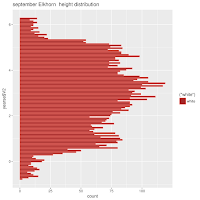
I like it.  It says something.  The tides of St Petersburg exhibit some interesting quirks, reflected in these plots.

These are Beeswarm plots, a kind of univariate distribution plot, like histograms, and maybe a stem and leaf diagram of a kind.  Each vertical plot represents a week (or so), hourly predictions for a quarter of the moon.  Black, for example, represents the span between New Moon and First Quarter. 

Maybe an inverted colorscheme would look better on the screen.  The following was inverted in The Gimp, and exported as PNG, from a PDF. 

Each vertical plot is a distribution plot, for one Quarter of a Lunar Cycle.  The first one, in January, represents the period between NM and First Quarter (Q1), then Q1-> FM; FM -> Q3; Q3->NM, etc.  48 of these quarters are represented, from January into December of 2019.

These are predictions, from Xtide.

I don't know why almost everywhere I graph a tide, no matter how, something interesting pops up!  What are we visualizing here?